ශුන්‍යයෙහි සමතාව

Empty balance scale
මෙම සංතුලන තුලාවේ කිරුම් තැටිවල වස්තූන් ශුන්‍යයක් අඩංගු වන්කේ, සම කෘණ්ඩ දෙකකට බෙදමිනි.
Listen to this article
(2 parts, 31 minutes)
  2. Part 2
Spoken Wikipedia icon
These audio files were created from a revision of this article dated
Error: no date provided
, and do not reflect subsequent edits.
(Audio help · More spoken articles)

ශුන්‍යය යනු ඉරට්ටේ සංඛ්‍යාවකි. වෙනත් වචන වලින් පැවසුවහොත් ශුන්‍යයෙහි සමත්වය; එනම් එය නිඛිලයක් වශයෙන් ඉරට්ටේද ඔත්තේද යන්න විමසුවහොත් එය ඉරට්ටේය. ශුන්‍යය යන්න ඉරට්ටේ බව සනාථ කිරීමට ඇති හොඳම ක්‍රමය වනුයේ "ඉරට්ටේ " සංඛ්‍යාවක අර්ථ දැක්වීම සලකා බැලීමෙනි. එනම් එය 2 හි ගුණිතයක් වන නිඛිලයකි. එනිසා ශුන්‍යය ඉරට්ටේ සංඛ්‍යාවක් දක්වන සියලු ලක්ෂණ පෙන්වයි; 0 ,2 න් බෙදිය හැකි වීම, 0 දෙපස ඇත්තේ ඔත්තේ සංඛ්‍යා වීම, සහ ශුන්‍යය කුලකයක් සමාන කුලක දෙකකට වෙන්කළ හැකි වීම ඒ අතර වෙයි.

සාමාන්‍ය ජනතාව‍ අතර ශුන්‍යයේ සමතාව පැටලිල්ලකට තුඩුදෙන කරුණකි. ප්‍රතිචාර කාලය භාවිතයෙන් කරන ලද පරීක්ෂණ වලදී බෙහෝ දෙනෙකුට "0" ඉරට්ටේ බව හඳුනාගැනීමට බොහෝ කාලයක් ගතවිය. සමහර සිසුන්, ගණිතඥයන් සහ සමහර ගුරුවරු "0" ඉරට්ටේ යයි පිලිනොගනී.

ශුන්‍යය ඉරට්ටේ වන්නේ ඇයි?

සම්මත අර්ථ දැක්වීමට අනුව ඉරට්ටේ සංඛ්‍යාවක අර්ථ දැක්වීම කෙලින්ම ශුන්‍යය ඉරට්ටේ බව සනාථ කරයි. සංඛ්‍යාවක් ඉරට්ටේ වීමට එය 2 හි නිඛිලමය ගුණාකාරයක් විය යුතුය. උදාහරණයක්‌ ලෙස 10 ඉරට්ටේ සංඛ්‍යාවක් වන්නේ 5×2 නිසාය. ඒ ආකාරයටම 0 ද 0×2 ආකාරයෙන් ලිවිය හැකි බැවින් ශුන්‍යය ඉරට්ටේ වෙයි.

මුලික පැහැදිලි කිරීම

වම් පස ඇති කොටුවල 0,2 සහ 4 වශයෙන් සුදු වස්තූන් ඇත ; දකුණු පස 1,3 සහ 5 බැගින් වස්තූන් , යුගල් නොවූ ඒවා රතු පාටින් දක්වා ඇත.
වස්තූන් 0 ඇති කොටුවෙහි රතුපාට වස්තූන් කිසිවක් ඉතිරිව නැත..

0 යනු සංඛ්‍යාවකි. සංඛ්‍යා යොදා ගන්නේ ගණනය කිරීම් සඳහාය. අවයව සහිත කුලකයක් ලබා දී ඇති විට යමෙකු සංඛ්‍යා යොදා ගන්නේ එම කුලකයේ තිබෙන අවයව ගණන ප්‍රකාශ කිරීමටයි. කිසිදු අවයවයක් නැත්නම් එය ශුන්‍ය ලෙස ගණන් කරයි. සමතාව යන සංකල්පය යොදා ගනු ලබන්නේ වස්තූන් දෙකක් සහිත කාණ්ඩ වෙන් කිරීම සඳහාය. යම්කිසි වස්තූන් සමුහයක් කිසිවක් ඉතිරි නැතිව දෙවරක් කාණ්ඩ වලට වෙන්කළ හැකිනම් එම වස්තූන් සමුහය ඉරට්ටේ වෙයි. නැත්නම් ඔත්තේ වෙයි.

මෙම අදහස වඩාත් සනාථ කිරීමට වස්තූන් යුගල් වශයෙන් ඇඳීම යොදාගත හැක. එවිට එක් වස්තුවක් ඉතිරි වෙයි නම් තිබුණ වස්තුන් ගණන ඔත්තේ වෙයි. නැත්නම් ඉරට්ටේ වෙයි. අභිශුනය කුලකය 2 හි ගුණාකාර සහිත කාණ්ඩ 0 කට වෙන්වෙයි. එනිසා 0 ඉරට්ටේ වෙයි. මේ සඳහා වන තවත් අර්ථ දැක්වීමක් ඇත. කාණ්ඩයක ඇති වස්තුන් සියල්ල එක සමාන නම් එය එක සමාන ගොඩවල් දෙකකට වෙන්කළ හැකිනම් එම වස්තුන් ගණන ඉරට්ටේ වෙයි.

සංඛ්‍යා, සංඛ්‍යා රේඛාවක නිරුපනය කල හැක. එවිට ඔත්තේ සහ ඉරට්ටේ සංඛ්‍යා එකිනෙකින් වෙන්කර හඳුනාගත හැක. ඔත්තේ සහ ඉරට්ටේ සංඛ්‍යා ඒකාන්තර වෙයි. ඕනෑම ඉරට්ටේ සංඛ්‍යාවකින් පටන් ගෙන ඉහලට හෝ පහලට දෙක බැගින් ගණන් කරගෙන ගිය විට අනෙකුත් ඉරට්ටේ සංඛ්‍යා හමුවේ. ඒ සඳහා 0 මග හැර යාමට අවශ්‍ය නැත.

Integers −4 through 10; even numbers are open circles; odd numbers are dots

ගුණකිරීම හඳුන්වාදීමත් සමඟ සමත්වය අංක ගණිතමය සමීකරණ මගින් අත්පත් කරගත හැක. සෑම නිඛිලයක්ම (2 × ▢) + 0 or (2 × ▢) + 1 ආකාර වෙයි. මුල් ඒවා ඉරට්ටේ වන අතර අනික්වා ඔත්තේ වෙයි.

සමතාවය පැහැදිලිකිරීම

ගණිතමය පදයක ඉතා නිවැරදි අර්ථ දැක්වීම බොහෝවිට සම්ප්‍රදායිකව පැවත එයි. සමහර ගණිතමය අර්ථ දැක්වීම් අපැහැදිලි තැන් ඉවත්වන සේ සකසා ඇත. ප්‍රථමක සංඛ්‍යා ඊට කදිම නිදසුනකි. 20 වන සියවසට පෙර ප්‍රථමක සංඛ්‍යාවක අර්ථ දැක්වීම අසන්තතික එකක් විය. ගොල්ද්බැච්, ලම්බර්ට්, ලෙජෙන්ද්රේ, කෙයිලි වැනි ගණිතඥයන් 1 ප්‍රථමක සංඛ්‍යාවක් ලෙස අර්ථ දැක්විය. නමුත් නවතම අර්ථ දැක්වීම අනුව "ප්‍රථමක සංඛ්‍යාවක්" යනු සාධක 2 ක් පමණක් ඇති ධන නිඛිලයකි. එනිසා 1 ප්‍රථමක නොවේ.

ශුන්‍යය අයත් නොවන පරිදි "ඉරට්ටේ සංඛ්‍යා " නැවත අර්ථ දැක්විය හැක. නමුත් එවිට ඉරට්ටේ සංඛ්‍යා අනුබධ්දයෙන් ඇති ප්‍රමේයයන් සනාථ කිරීම අපහසු වෙයි. දැනටමත් එහි බලපෑම ඔත්තේ සහ ඉරට්ටේ සංඛ්‍යා ආශ්‍රිතව ඇති වීජ ගණිතය මය නීති සඳහා එල්ල වී හමාරය. මේ සඳහා බොහොමයක් නීති එකතු කිරීම, අඩු කිරීම සහ ගුණ කිරීම කෙරෙහි අවධානය යොමු කරයි. 

  ඉරට්ටේ  ±  ඉරට්ටේ =  ඉරට්ටේ
   ඔත්තේ  ± ඔත්තේ  =  ඉරට්ටේ
   ඉරට්ටේ × නිඛිලයක්  =  ඉරට්ටේ

මේ සඳහා අගයන් ආදේශ කල විට පහත පරිදි පිළිතුරු ලැබේ. 


   2 − 2 = 0
   −3 + 3 = 0
   4 × 0 = 0

ශුන්‍යය ඉරට්ටේ නොවුනහොත් ඉහත නීති සත්‍ය නොවේ. අඩුම වශයෙන් ඒවා වෙනස් කිරීමට හෝ සිදු වෙයි. උදාහරණයක් ලෙස එක්තරා අධ්‍යනයක් පෙන්වා දෙන්නේ ඉරට්ටේ සංඛ්‍යා 2 හි නිඛිලමය ගුණාකාර ලෙස අර්ථ දැක්විය හැකි බවයි. නමුත් 0 ඔත්තේ හෝ ඉරට්ටේ නොවේ. මේ අනුව එම අධ්‍යනය සම්මතයෙන් බැහැර වන අවස්ථා ද ඇත. 

   ඉරට්ටේ ±ඉරට්ටේ= ඉරට්ටේ (හෝ 0 )
   ඔත්තේ ± ඔත්තේ = ඉරට්ටේ (හෝ 0)
   ඉරට්ටේ × ශුන්‍යය නොවන ඕනෑම නිඛිලයක් = ඉරට්ටේ

ගණිතමය සම්බන්ධය

සංඛ්‍යා පිලිබඳ නොයෙකුත් ඉගැන්වීම් වලින් ලැබෙන පිළිතුරු වීජ ගණිමය සහ අංක ගණිතමය වශයෙන් ඉරට්ටේ සංඛ්‍යාවක ලක්ෂණ පෙන්වයි. උදාහරනයක් ලෙස ධන සංඛ්‍යා සඳහා ආවේනික සාධක ඇති බව සැලකීමෙන් පෙනී යන්නේ යමෙකුට යම් සංඛ්‍යාවක් සැලකීමෙන් එයට ඔත්තේ හෝ ඉරට්ටේ ප්‍රථමක සාධක කියක් ඇත්දැයි නිශ්චය කල හැකි බවයි..

ඔත්තේ නොවීම

n නැමති සංඛ්‍යාව ඔත්තේ වීමට k නිඛිලයක් විට n=2k +1 විය යුතුය. 0 ඔත්තේ නොවේ යයි සාධනය කිරීමට ඇති එක් ක්‍රමයක් නම් විසංවාදී සාධන ක්‍රමයයි. 0 =2k +1 නම් k=-1/2 වෙයි. එය නිඛිලයක් නොවේ. 0 ඔත්තේ නොවන නිසා නොදන්නා සංඛ්‍යාවක් ඔත්තේ යයි ඔප්පු කල විට එය 0 විය නොහැක. ප්‍රස්ථාර ආශ්‍රිත ගණනය කිරීම් සලකා බැලීමේදී පෙනී යන්නේ ඔත්තේ බල සහිත ප්‍රස්ථාර සඳහා අවම වශයෙන් එක් ඉරට්ටේ ශීර්ෂයක් වත් ඇති බවයි. සාමාන්‍යයෙන් ඔත්තේ ශීර්ෂයන් සහිත ඉරට්ටේ ප්‍රස්ථාර බල එකතු කිරීමේ ප්‍රස්ථාර මගින් දැක්වෙයි.

ඉරට්ටේ සහ ඔත්තේ අගයන් අන්තර් හුවමාරු වීම

0->1->2->3->4->5->6->... in alternating colors
ස්වභාවික සංඛ්‍යා පුනරාවර්තනය වීම.

0 ඉරට්ටේ සංඛ්‍යාවක් වීම සහ ඔත්තේ සහ ඉරට්ටේ සංඛ්‍යා එකිනෙක හුවමාරු වීම අනෙකුත් සියළු සංඛ්‍යා වල සමතාවය අධ්‍යනය කිරීමට ප්‍රමාණවත් වෙයි. මෙම අධ්‍යනය ස්වභාවික ඉරට්ටේ සංඛ්‍යා වල පුනරාවර්තන අර්ථ දැක්වීමකට යොදා ගැනීමට පුළුවන. 

   0 ඔත්තේ වෙයි 
   (n + 1) n ඉරට්ටේ නොවේ නම් පමණක් n+1 ඉරට්ටේ වෙයි.

මෙම අර්ථ දැක්වීම් සංකල්පිත වාසියක් ගෙනදෙයි. එනම් එය ස්වභාවික සංඛ්‍යා වල පැවැත්ම සමඟ ඉතා සුළු වශයෙන් බැදේ.

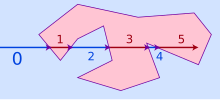
Non-convex polygon penetrated by an arrow, labeled 0 on the outside, 1 on the inside, 2 on the outside, etc.
බහු ආශ්‍රයක ශීර්ෂ පරීක්ෂාව

පරිගණක ජ්‍යාමිතියේ එන සම්ප්‍රදායික බහු අශ්‍රයක ශීර්ෂ පරික්ෂාව ඉහත ප්‍රකාශ සනාථ කරයි. ශීර්ෂයක් බහු ආශ්‍රයක් තුල පවතීද යන්න සොයා බැලීම සඳහා අනන්තයේ සිට පැමිණෙන ආලෝක ධාරාවක් එම ශීර්ෂය හරහා යවා එය බහු ආශ්‍රයේ ශීර්ෂ හරහා ගමන් කරයි දැයි බැලිය යුතුය. අදාළ ශීර්ෂය බහුඅශ්‍රයෙන් පිටත වේ නම් එම කැපෙන අවස්ථා ගණන ඉරට්ටේ වෙයි.



A graph with 9 vertices, alternating colors, labeled by distance from the vertex on the left
ද්වේශාක වක්‍රය නිර්මාණය කිරීම

ප්‍රස්තාර සැලකීමේදී ද්වීශාක ප්‍රස්ථාරයක් යනු ශීර්ෂ එකිනෙකට වෙනස් වර්ණ වලින් සමන්විත වූ ප්‍රස්තරයකි. එහි අනුයාත ශීර්ෂ එකිනෙකට වෙනස් වර්ණ දරයි. සම්බන්ධිත ප්‍රස්ථාරයක ඔත්තේ වක්‍ර නැත්නම් එක් ශීර්ෂයක් තෝරාගෙන එය මුලික ශීර්ෂය ලෙස සලකා අනුයාත ශීර්ෂ කළු සහ සුදු වර්ණ ගැන්වීමෙන් මෙය කල හැක.

වීජ ගණිතමය රටාවන්

Integers −4 through +4 arranged in a corkscrew, with a straight line running through the evens
2Z (නිල්) Z හි උප කාණ්ඩයක් ලෙස

විජ ගණිතය ට අනුව ඉරට්ටේ නිඛිල විවිධ වීජ ගණිතමය ව්‍යූහයන් 0 අවශ්‍ය වන පරිදි නිර්මාණය කරයි. ඉලක්කම් දෙකක් කෙසේ එකතු කලද (ශුන්‍යය සහිතව) පිළිතුර ඉරට්ටේ වීම, ඉරට්ටේ සංඛ්‍යා වල විලෝමය එකතුකළ විට පිළිතුර ශුන්‍ය වීම සහ ආකලනය න්‍යාදේශ වීම ඉරට්ටේ නිඛිල එකම කාණ්ඩයකට අයත් බව සනාථ කරයි. තව දුරටත් සලකා බැලූ විට ආකලනය යටතේ ඉරට්ටේ නිඛිල කාණ්ඩය සම්පුර්ණ නිඛිල කාණ්ඩයේ උප කාණ්ඩයක් වෙයි.

"ඉරට්ටේ ×ඕනෑම නිඛිලයක්=ඉරට්ටේ" යන නිතිය සනාථ කරන්නේ නිඛිල වල ඒවා සුවිශේෂ ලක්ෂණ දක්වන බවයි. විශේෂයෙන් සැලකු විට ඉරට්ටේ නිඛිල යනු k නිඛිලයක් විට k≡ 0 (ශේෂය 2). මෙය බහුපද ශ්‍රිත අධ්‍යනයේදී වැදගත් වෙයි.

අධ්‍යනය

Bar chart; see description in body text
එක් එක් වසර වල ළමුන්ගේ පිළිතුරු ප්‍රතිශතයක් ලෙස.

මෙම විෂය පථය ප්‍රාථමික අධ්‍යාපනයේ ප්‍රථම වසර දෙක හෝ තුන ඔත්තේ සහ ඉරට්ටේ සංඛ්‍යා පිළිබඳව ඉගැන්වීමේදී අවධානයට යොමු වෙයි.

ළමුන්ගේ දැනුම

දකුණු පසින් දක්වා ඇති වගුව 1 වසරේ සිට 6 වසර දක්වා ඉංග්‍රීසි අධ්‍යාපනය ලබන සිසුන්ගෙන් මේ ගැන විමසූ විට ලැබුණ පිළිතුරු දක්වයි. මෙම දත්ත ලබා ගෙන ඇත්තේ ලෙන් ෆොර්බිෂර් මහතා විසින් සිදු කරන ලද පරීක්ෂණයක් ඇසුරෙනි. එක්තරා ප්‍රථමික පරීක්ෂණයක් වයස අවුරුදු 7 සිසුන් 400 යොදා ගෙන සිදුකළ අතර එහිදී 45% සිසුන් ශුන්‍යය හි සමතාවය විමසු විට ඔත්තෙට වඩා ඉරට්ටේ තෙරුහ. තවත් පරීක්ෂනයක දී ඔවුන්ට පිළිතුරු වශයෙන් "දෙකෙන් එකක් වත් නොවේ", "දෙකම නිවැරදි" සහ "නොදනිමි" යන අවස්ථා ලබා දුන් විට එම ප්‍රතිශතය 32% දක්වා පහත වැටුණි. ශුන්‍යය ඉරට්ටේ බව සෙවීමේ පරීක්ෂණ වල ප්‍රථිපල 3 වසරේ සිට 6 වසර දක්වා බැලූ කල 50% පමණ වේ. සම්මුඛ පරීක්ෂණ වලදී ලැබුණ ප්‍රථිපල මෙසේ වෙයි. එක් 5 වසර සිසුවෙකු පැවසුවේ ශුන්‍යය 2 ගුණාකාර වගුවේ තිබුණ නිසා එය ඉරට්ටේ වන බවයි. 4 වසර සිසුන් පිරිසක් පැවසුවේ එය සමාන ගොඩවල් වලට බෙදිය හැකි බවයි.

ගුරුවරුන්ගේ දැනුම

ගුරුවරුන්ගේ දැනුම මැනීම සඳහා මිචිගන් විශ්වවිද්‍යාලයේ ගණිත අධ්‍යනය පිලිබඳ පර්යේෂකයෝ පිරිසක් "0 ඉරට්ටේ වෙයි" යන්න ප්‍රකාශ කර එහි සත්‍ය අසත්‍ය බව විමසීම සඳහා ප්‍රශ්න 250 කින් පමණ සමන්විත ප්‍රශ්නාවලියක් ගුරුවරුන් හට ඉදිරිපත් කරන ලදී. ඇමරිකා එක්සත් ජනපදයේ ප්‍රථමික ගුරුවරු 700 පමණ සහභාගී කරගෙන 2000-2004 වර්ෂ වල කරන ලද සමීක්ෂණයකින් පැහැදිලි කරගත්තේ ළමුන් වෙනුවෙන් කල පරීක්ෂණ වල සාර්ථක බව එයින් වැඩි වන බවයි. 2008 වර්ෂයේදී සිදු කරන ලද තවත් පරීක්ෂණයකින් අනාවරණය වුයේ එක්තරා පාසලක ගුරුවරු සියළු දෙනා ශුන්‍යය ඔත්තේ හෝ ඉරට්ටේ යන කොට්ටාශ දෙකටම අයත් නොවන බවට උගන්වා ඇති බවයි.

ඉගැන්වීමේදී අවධානයට ගතයුතු කරුණු

ගණිතමය වශයෙන් ශුන්‍යය ඉරට්ටේ බව ඔප්පු කිරීම අර්ථ දැක්වීමක් හා සැසදීමක් පමණි. නමුත් අධ්‍යනයේදී ඊට වඩා සංකීර්ණ පැහැදිලි කිරීමක් අවශ්‍ය වෙයි. ඔප්පු කිරීමේදී හටගන්නා එක්තරා ගැටලුවක් වන්නේ අර්ථ දැක්වීමේ ඇති "ඉරට්ටේ" යනු "2 හි නිඛිලමය ගුණාකාර" යන්න සෑම අවස්ථාවකටම සුදුසු නොවීමයි. මන්ද යත් ප්‍රථමික අධ්‍යාපනයේ පළමු වසර කිහිපයේ ඉගෙන ගන්නා ළමුන් "නිඛිල", "ගුණාකාර" වැනි සංකල්ප නොදන්න නිසා සහ 0 ගුණ කිරීමට නොදන්නා බැවිනි.

නිතිපතා පද සම්බන්ධය

0 සහිත සම්බන්ධතා බොහෝ වෙලාවට උපමා සහිත අර්ථ නිරුපනයක් එකතු කරයි. මෙවැනි ගැටළු සහිත තත්වයන් අන්තර්ජාල පරිශීලකයන් වෙත යොමු කර විට බොහොමයක් වෙබ් අඩවි වල සාකච්චාවට භාජනය විය. ශුන්‍යය ඔත්තේ හෝ ඉරට්ටේ නොවේ යන මතය දරන බොහොමයක් දෙනා ශුන්‍යයෙහි සමතාවය සෑම නිතියකටම ප්‍රතිවිරුද්ධ නිදර්ශනයක් ඇති බව සනාථ කිරීම සඳහා යොදා ගනී.

"ඔතතේ සහ ඉරට්ටේ" ක්‍රීඩාවද මෙයින් බලපෑමකට හසුවිය : ක්‍රීඩකයන් දෙදෙනාම ඇඟිලි 0 යොමු කළහොත් සියළු ඇඟිලි ගණන 0 වේ. එක් ගුරුවරයෙක් පවසා සිටියේ මෙම ක්‍රීඩාවෙහි නිරතවීම කුඩා දරුවන්ට 0, දෙකෙන් බෙදෙන බව පෙන්වීමට යොදාගත හැකි යයි පවසයි.

References

Notes

Bibliography

විකිඋද්ධෘත සතුව පහත තේමාව සම්බන්ධයෙන් උද්ධෘත එකතුවක් ඇත: