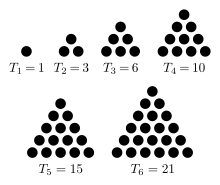
The first six triangular numbers A triangular number is a number that is the sum of all of the natural numbers up to a certain number. When formed using regularly spaced dots, they tend to form a shape of either an equilateral or a right triangle , hence the name.[ 1]
For example, 10 is a "triangular number" because
10
=
1
+
2
+
3
+
4
{\displaystyle 10=1+2+3+4}
The first 25 triangular numbers are: 0, 1, 3, 6, 10, 15, 21, 28, 36, 45, 55, 66, 78, 91, 105, 120, 136, 153, 171, 190, 210, 231, 253, 276, 300, 325, 351, and so on.
A triangular number is calculated by the equation:
n
(
n
+
1
)
2
{\displaystyle {\frac {n(n+1)}{2}
References
Other polynomial numbers
Hilbert
Idoneal
Leyland
Loeschian
Lucky numbers of Euler
Fibonacci Jacobsthal
Leonardo
Lucas
Padovan
Pell
Perrin
Possessing a specific set of other numbers
Congruent
Knödel
Riesel
Sierpiński
Expressible via specific sums
Nonhypotenuse
Polite
Practical
Primary pseudoperfect
Ulam
Wolstenholme
Figurate numbers
2-dimensional
centered
Centered triangular
Centered square
Centered pentagonal
Centered hexagonal
Centered heptagonal
Centered octagonal
Centered nonagonal
Centered decagonal
Star non-centered
Triangular Square Square triangular
Pentagonal
Hexagonal
Heptagonal
Octagonal
Nonagonal
Decagonal
Dodecagonal
3-dimensional
centered
Centered tetrahedral
Centered cube
Centered octahedral
Centered dodecahedral
Centered icosahedral non-centered
Tetrahedral
Cubic Octahedral
Dodecahedral
Icosahedral
Stella octangula pyramidal
4-dimensional
non-centered
Pentatope
Squared triangular
Tesseractic
Combinatorial numbers
Bell
Cake
Catalan
Dedekind
Delannoy
Euler
Eulerian
Fuss–Catalan
Lah
Lazy caterer's sequence
Lobb
Motzkin
Narayana
Ordered Bell
Schröder
Schröder–Hipparchus
Stirling first
Stirling second
Wieferich
Wall–Sun–Sun
Wolstenholme prime
Wilson
Pseudoprimes
Carmichael number Catalan pseudoprime
Elliptic pseudoprime
Euler pseudoprime
Euler–Jacobi pseudoprime
Fermat pseudoprime
Frobenius pseudoprime
Lucas pseudoprime
Lucas–Carmichael number
Somer–Lucas pseudoprime
Strong pseudoprime
Arithmetic functions and dynamics
Blum
Cyclic
Erdős–Nicolas
Erdős–Woods
Friendly
Giuga
Harmonic divisor
Lucas–Carmichael
Pronic
Regular
Rough
Smooth
Sphenic
Størmer
Super-Poulet
Zeisel
Arithmetic functions Digit sum
Digit sum
Digital root
Self
Sum-product Digit product
Multiplicative digital root
Sum-product Coding-related Other
Dudeney
Factorion
Kaprekar
Kaprekar's constant
Keith
Lychrel
Narcissistic
Perfect digit-to-digit invariant
Perfect digital invariant
P-adic numbers -relatedDigit -composition related
Palindromic
Pandigital
Repdigit Repunit Self-descriptive
Smarandache–Wellin
Strictly non-palindromic
Undulating Digit-permutation related
Cyclic
Digit-reassembly
Parasitic
Primeval
Transposable Divisor-related
Equidigital
Extravagant
Frugal
Harshad Polydivisible
Smith
Vampire Other
Pancake number
Sorting number