截角八面體堆砌
| 截角八面體堆砌 | |
|---|---|
 | |
| 類型 | 均勻堆砌 |
| 維度 | 3 |
| 對偶多胞形 | 鍥形四面體堆砌 |
| 數學表示法 | |
| 考克斯特符號 | |
| 纖維流形記號 | 8o:2 |
| 施萊夫利符號 | 2t{4,3,4} t1,2{4,3,4} |
| 性質 | |
| 胞 | (4.6.6) |
| 面 | {4} {6} |
| 組成與佈局 | |
| 棱圖 | {3} 等腰三角形 |
| 顶点图 | 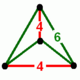 鍥形四面體 |
| 對稱性 | |
| 對稱群 | , [4,3,4] |
| 空間群 | Im3m (229) |
| 考克斯特群 | [[4,3,4]] |
| 特性 | |
| 顶点正 | |
在幾何學中,截角八面體堆砌又稱為克爾文結構是三維空間內28個半正密鋪之一,由截角八面體獨立堆積而成,雖然他每個胞都全等、每邊皆等長,但其不能稱為正密鋪,因為雖然它只由一種胞,截角八面體組成,但是該胞不是正多面體,因此並非所有“面”皆全等,因此截角八面體堆砌只能稱為半正堆砌。截角八面體堆砌曾出現於克爾文的研究中,克爾文指出,這種結構構成的泡沫結構可能是表面積最小的理想泡沫結構,然而1993年時物理學家丹尼斯·韋爾和羅伯特·費倫指出存在表面積更小的韋爾—費倫結構。[1]
性質

在截角八面體堆砌中,每個頂點周圍皆有有四個截角八面體,且全由截角八面體組成,因此其胞可遞。它也存在邊可遞的特性,由於其具有2個六邊形和一個正方形,且截角八面體堆砌的每個頂點都是4個截角八面體的公共頂點,因此每條邊和頂點也存在點可遞的特性。
截角八面體堆砌可以被視為體心立方晶格的沃羅諾伊圖。開爾文男爵推測截角八面體堆砌若將其面和邊彎曲且保留原來的布局將會變為最佳的肥皂泡沫理想結構[2]。而在1993年發現韋爾—費倫結構是比截角八面體堆砌更佳的泡沫結構。
命名
康威稱截角八面體堆砌為truncated octahedrille[3],在他的建築學和反射的細分列表,與其對偶合稱oblate tetrahedrille又稱為鍥形四面體堆砌。雖然正四面體不能單獨填充整個空間,但截角八面體堆砌的對偶具有相同的鍥形四面體胞與等腰三角形的面。
此外由於截角八面體堆砌的每個頂點都是4個截角八面體的公共頂點,因此也可稱為四階截角八面體堆砌。
歷史
克耳文1887年提出了一個問題:如何將空間劃分為等一系列體積的胞,且每個胞的表面積最小。簡而言之,效率最高的泡沫結構是什麼?[2]這個問題被稱為克耳文問題。
克耳文提出了一種基於截角八面體堆砌的泡沫結構,因此截角八面體堆砌又被稱為克耳文結構。截角八面體堆砌是一種由截角八面體獨立填滿三維空間的幾何結構,是凸均勻堆砌體的一種,其中截角八面體是一個空間填充十四面體,由6個正方形面和8個正六邊形面組成。為了使其符合泡沫的經驗定律普拉托定律[4][5],克耳文結構中的截角八面體之六邊形面有略微彎曲。不過這個被認為是最佳的泡沫結構於100年後才發現反例,該反例為韋爾—費倫結構[1],其表面積比克耳文結構還要小0.3%。2009年,魯格羅・加布里埃利(Ruggero Gabbrielli)[6]發表了一種使用斯威夫特–奧昂貝格方程在最小曲面上找到克耳文問題候選解的方法。[7][8]
對稱性與表面塗色
| 空間群 | Im3m (229) | Pm3m (221) | Fm3m (225) | F43m (216) | Fd3m (227) |
|---|---|---|---|---|---|
| 纖維流形 | 8o:2 | 4−:2 | 2−:2 | 1o:2 | 2+:2 |
| 考克斯特群 | ×2 [[4,3,4]] =[4[3[4]]] |
[4,3,4] =[2[3[4]]] |
[4,31,1] =<[3[4]]> |
[3[4]] |
×2 [[3[4]]] =[[3[4]]] |
| 考克斯特符號 | |||||
| 截角八面體 | 1 |
1:1 |
2:1:1 |
1:1:1:1 |
1:1 |
| 頂點圖 | 
|

|

|
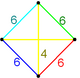
|
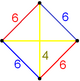
|
| 頂點 值 對稱性 |
[2+,4] (order 8) |
[2] (order 4) |
[ ] (order 2) |
[ ]+ (order 1) |
[2]+ (order 2) |
| 圖像 表面依胞 上色 |

|

|

|

|

|
相關多面體和鑲嵌

考克斯特群[4,3,4]、![]()
![]()
![]()
![]()
![]()
![]()
![]() 產生15個排列均勻的鑲嵌中,9個具有獨特的的幾何形狀,包括交錯立方体堆砌、擴展立方堆砌是幾何上相同的立方體堆砌。
產生15個排列均勻的鑲嵌中,9個具有獨特的的幾何形狀,包括交錯立方体堆砌、擴展立方堆砌是幾何上相同的立方體堆砌。
| 空間群 | 纖維流形 | 擴展 對稱群 |
擴展 标记 |
阶 | 蜂巢體 (堆砌) |
|---|---|---|---|---|---|
| Pm3m (221) |
4−:2 | [4,3,4] | ×1 | ||
| Fm3m (225) |
2−:2 | [1+,4,3,4] ↔ [4,31,1] |
↔ |
Half | |
| I43m (217) |
4o:2 | [[(4,3,4,2+)]] | Half × 2 | ||
| Fd3m (227) |
2+:2 | [[1+,4,3,4,1+]] ↔ [[3[4]]] |
↔ |
Quarter × 2 | |
| Im3m (229) |
8o:2 | [[4,3,4]] | ×2 |
|
考克斯特群[4,31,1], ![]()
![]()
![]()
![]()
![]() , 考克斯特群產生 9個排列均勻的鑲嵌中,其中4個具有獨特的的幾何形狀,包括交替立方体堆砌。
, 考克斯特群產生 9個排列均勻的鑲嵌中,其中4個具有獨特的的幾何形狀,包括交替立方体堆砌。
| 空間群 | 纖維流形 | 擴展 對稱群 |
擴展 标记 |
阶 | 蜂巢體 (堆砌) |
|---|---|---|---|---|---|
| Fm3m (225) |
2−:2 | [4,31,1] ↔ [4,3,4,1+] |
↔ |
×1 | |
| Fm3m (225) |
2−:2 | <[1+,4,31,1]> ↔ <[3[4]]> |
↔ |
×2 | |
| Pm3m (221) |
4−:2 | <[4,31,1]> | ×2 |
|
立方體堆砌是考克斯特群中的五個結構特別的均勻堆砌[9]之一,其對稱性可以乘以環在考克斯特-迪肯符號的對稱性:
| 空間群 | 纖維流形 | 方形 對稱群 |
擴展 對稱群 |
擴展 标记 |
擴展 阶 |
蜂巢體 (堆砌) |
|---|---|---|---|---|---|---|
| F43m (216) |
1o:2 | a1 | [3[4]] | ×1 | (None) | |
| Fd3m (227) |
2+:2 | p2 | [[3[4]]] | ↔ |
×2 | |
| Fm3m (225) |
2−:2 | d2 | <[3[4]]> ↔ [4,3,31,1] |
↔ |
×2 | |
| Pm3m (221) |
4−:2 | d4 | [2[3[4]]] ↔ [4,3,4] |
↔ |
×4 | |
| Im3m (229) |
8o:2 | r8 | [4[3[4]]] ↔ [[4,3,4]] |
↔ |
×8 |
交錯形式
截角八面體堆砌可以交錯,從截角八面體的空隙中創建不規則四面體單元建立正二十面體。有三個相關的結構對應三種考克斯特—迪肯符號:![]()
![]()
![]()
![]()
![]()
![]()
![]() 、
、![]()
![]()
![]()
![]()
![]() 和
和![]()
![]()
![]()
![]()
![]() ,且有對稱性[4,3+,4]、[4,(31,1)+]和[3[4]]+。第一個和最後一個的對稱性為[[4,3+,4]] and [[3[4]]]+的一倍。
,且有對稱性[4,3+,4]、[4,(31,1)+]和[3[4]]+。第一個和最後一個的對稱性為[[4,3+,4]] and [[3[4]]]+的一倍。
截角八面體堆砌可以當作在α-rhombihedral晶體內的硼原子位置,在二十面體的中心是面心立方晶格的位置。[10]
| 空間群 | I3 (204) | Pm3 (200) | Fm3 (202) | Fd3 (203) | F23 (196) |
|---|---|---|---|---|---|
| 纖維流形 | 8−o | 4− | 2− | 2o+ | 1o |
| 考克斯特群 | [[4,3+,4]] | [4,3+,4] | [4,(31,1)+] | [[3[4]]]+ | [3[4]]+ |
| 考克斯特符號 | |||||
| 階 | 二 | 全 | 半 | 四分之一 二 |
四分之一 |
折疊投影
| 考克斯特群 | ||
|---|---|---|
| 考克斯特記號 | ||
| 形狀 |  過截角正方體堆砌 |
 截角正方形鑲嵌 |
參見
参考文獻
- ^ 1.0 1.1 Wearie-Phelan Bubbles. steelpillow.com. [2019-09-29]. (原始内容存档于2019-08-06).
- ^ 2.0 2.1 Lord Kelvin (Sir William Thomson), On the Division of Space with Minimum Partitional Area (PDF), Philosophical Magazine, 1887, 24 (151): 503 [2019-09-29], doi:10.1080/14786448708628135, (原始内容 (PDF)存档于2021-11-26).
- ^ John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN 978-1-56881-220-5 (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, Architectonic and Catoptric tessellations, p 292-298, includes all the nonprismatic forms)
- ^ Jean E. Taylor. "The Structure of Singularities in Soap-Bubble-Like and Soap-Film-Like Minimal Surfaces". Annals of Mathematics, 2nd Ser., Vol. 103, No. 3. May, 1976, pp. 489–539.
- ^ Frederick J. Almgren Jr and Jean E. Taylor, “The geometry of soap films and soap bubbles”, Scientific American, vol. 235, pp. 82–93, July 1976.
- ^ Gabbrielli, Ruggero. Ruggero Gabbrielli - Google Scholar Citations. scholar.google.com.
- ^ Gabbrielli, Ruggero. A new counter-example to Kelvin's conjecture on minimal surfaces. Philosophical Magazine Letters. 2009-08-01, 89 (8): 483–491. ISSN 0950-0839. doi:10.1080/09500830903022651.
- ^ Freiberger, Marianne. Kelvin's bubble burst again | plus.maths.org. Plus Magazine (University of Cambridge). 2009-09-24 [2017-07-04]. (原始内容存档于2019-09-29) (英语).
- ^ [1] (页面存档备份,存于互联网档案馆), A000029 (页面存档备份,存于互联网档案馆) 6-1 cases, skipping one with zero marks
- ^ Williams, Robert. The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. 1979. ISBN 0-486-23729-X., p 199, Figure 5-38.
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN 978-1-56881-220-5 (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, Architectonic and Catoptric tessellations, p 292-298, includes all the nonprismatic forms)
- George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) (Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs)
- Branko Grünbaum, Uniform tilings of 3-space. Geombinatorics 4(1994), 49 - 56.
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [2] (页面存档备份,存于互联网档案馆)
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10] (1.9 Uniform space-fillings)
- A. Andreini, Sulle reti di poliedri regolari e semiregolari e sulle corrispondenti reti correlative (On the regular and semiregular nets of polyhedra and on the corresponding correlative nets), Mem. Società Italiana della Scienze, Ser.3, 14 (1905) 75–129.
外部連結
- Klitzing, Richard. 3D Euclidean Honeycombs o4x3x4o - batch - O16. bendwavy.org.
- Uniform Honeycombs in 3-Space: 05-Batch (页面存档备份,存于互联网档案馆)
- 埃里克·韦斯坦因. Space-filling polyhedron. MathWorld.





