توابع معکوس مثلثاتی در ریاضیات ، معکوس تابعهای مثلثاتیاند که طبق تعریف تابع وارون، بُرد آنها زیرمجموعهٔ دامنهٔ تابع اصلی دیگری است. از آنجایی که تابعهای مثلثاتی هیچکدام یک به یک نیستند، برای همین برای اینکه وارون آنها تابع بماند (به ازای یک ورودی چند خروجی به دست نیاید) باید آنها را محدود کرد (نگاه کنید به آزمون خط افقی).
برای نمونه اگر تعریف کنیم
y
=
arcsin
(
x
)
{\displaystyle y=\operatorname {arcsin} (x)}
y
=
s
i
n
−
1
x
{\displaystyle y=sin^{-1}x}
x
=
sin
(
y
)
{\displaystyle x=\operatorname {sin} (y)}
x یکتا میتوان چندین y پیدا کرد که به ازای آن
x
=
sin
(
y
)
{\displaystyle x=\operatorname {sin} (y)}
y مساوی صفر، π و ۲π که به ازای همهٔ آنها مقدار سینوس یا x برابر با صفر است و این به این معنی است که تابع وارون سینوس یا arcsin یا
s
i
n
−
1
x
{\displaystyle sin^{-1}x}
arcsin
(
0
)
=
0
,
π
,
2
π
{\displaystyle \operatorname {arcsin} (0)=0,\pi ,2\pi }
بُرد یا خروجی قرار میدهیم تا به ازای یک ورودی چندین خروجی نداشته باشند.
نمایش توابع معکوس مثلثاتی به فرم مشابه
s
i
n
−
1
x
{\displaystyle sin^{-1}x}
جان هرشل در سال ۱۸۱۳ به کار برده شد. این فرم را نباید با مقدار
1
sin
x
{\displaystyle {\operatorname {1} \over \operatorname {sin} \!x}
y
=
f
−
1
(
x
)
{\displaystyle y=f^{-1}(x)}
همچنین Arc به معنای "قوس" یا کمانی است که مقدار نسبت مثلثاتی آن معلوم است.
تابعهای اصلی در جدول زیر آورده شدهاند:
برخی تعاریف:
آرکسینوس (سینوساینورس) یک عدد مفروض زاویهای است که سینوس آن برابر آن عدد مفروض است
آرککسینوس (کسینوساینورس) یک عدد مفروض زاویهای است که کسینوس آن برابر آن عدد مفروض است
آرکتانژانت (تانژانتاینورس) یک عدد مفروض زاویهای است که تانژانت آن برابر آن عدد مفروض است
آرککتانژانت (کتانژانتاینورس) یک عدد مفروض زاویهای است که کتانژانت آن برابر آن عدد مفروض است.[ ۱]
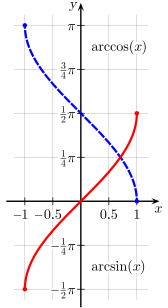
نمودار تابع های
arcsin
(
x
)
{\displaystyle \operatorname {arcsin} (x)}
arccos
(
x
)
{\displaystyle \operatorname {arccos} (x)}
نمودار تابع های
arctan
(
x
)
{\displaystyle \operatorname {arctan} (x)}
arccot
(
x
)
{\displaystyle \operatorname {arccot} (x)}
نمودار تابع های
arcsec
(
x
)
{\displaystyle \operatorname {arcsec} (x)}
arccsc
(
x
)
{\displaystyle \operatorname {arccsc} (x)}
زاویههای مکمل:
arccos
x
=
π
2
−
arcsin
x
{\displaystyle \arccos x={\frac {\pi }{2}-\arcsin x}
arccot
x
=
π
2
−
arctan
x
{\displaystyle \operatorname {arccot} x={\frac {\pi }{2}-\arctan x}
arccsc
x
=
π
2
−
arcsec
x
{\displaystyle \operatorname {arccsc} x={\frac {\pi }{2}-\operatorname {arcsec} x}
ورودیهای با علامت مخالف:
arcsin
(
−
x
)
=
−
arcsin
x
{\displaystyle \arcsin(-x)=-\arcsin x\!}
arccos
(
−
x
)
=
π
−
arccos
x
{\displaystyle \arccos(-x)=\pi -\arccos x\!}
arctan
(
−
x
)
=
−
arctan
x
{\displaystyle \arctan(-x)=-\arctan x\!}
arccot
(
−
x
)
=
π
−
arccot
x
{\displaystyle \operatorname {arccot}(-x)=\pi -\operatorname {arccot} x\!}
arcsec
(
−
x
)
=
π
−
arcsec
x
{\displaystyle \operatorname {arcsec}(-x)=\pi -\operatorname {arcsec} x\!}
arccsc
(
−
x
)
=
−
arccsc
x
{\displaystyle \operatorname {arccsc}(-x)=-\operatorname {arccsc} x\!}
ورودیهای وارون شده:
arccos
(
1
/
x
)
=
arcsec
x
{\displaystyle \arccos(1/x)\,=\operatorname {arcsec} x\,}
arcsin
(
1
/
x
)
=
arccsc
x
{\displaystyle \arcsin(1/x)\,=\operatorname {arccsc} x\,}
arctan
(
1
/
x
)
=
1
2
π
−
arctan
x
=
arccot
x
,
if
x
>
0
{\displaystyle \arctan(1/x)={\tfrac {1}{2}\pi -\arctan x=\operatorname {arccot} x,{\text{ if }x>0\,}
arctan
(
1
/
x
)
=
−
1
2
π
−
arctan
x
=
−
π
+
arccot
x
,
if
x
<
0
{\displaystyle \arctan(1/x)=-{\tfrac {1}{2}\pi -\arctan x=-\pi +\operatorname {arccot} x,{\text{ if }x<0\,}
arccot
(
1
/
x
)
=
1
2
π
−
arccot
x
=
arctan
x
,
if
x
>
0
{\displaystyle \operatorname {arccot}(1/x)={\tfrac {1}{2}\pi -\operatorname {arccot} x=\arctan x,{\text{ if }x>0\,}
arccot
(
1
/
x
)
=
3
2
π
−
arccot
x
=
π
+
arctan
x
,
if
x
<
0
{\displaystyle \operatorname {arccot}(1/x)={\tfrac {3}{2}\pi -\operatorname {arccot} x=\pi +\arctan x,{\text{ if }x<0\,}
arcsec
(
1
/
x
)
=
arccos
x
{\displaystyle \operatorname {arcsec}(1/x)=\arccos x\,}
arccsc
(
1
/
x
)
=
arcsin
x
{\displaystyle \operatorname {arccsc}(1/x)=\arcsin x\,}
در صورتی که تنها بخشی از جدول سینوس را داشته باشیم:
arccos
x
=
arcsin
1
−
x
2
,
if
0
≤
x
≤
1
{\displaystyle \arccos x=\arcsin {\sqrt {1-x^{2},{\text{ if }0\leq x\leq 1}
arctan
x
=
arcsin
x
x
2
+
1
{\displaystyle \arctan x=\arcsin {\frac {x}{\sqrt {x^{2}+1}
هرگاه از ریشهٔ دوم یک عدد مختلط استفاده شد، باید ریشهٔ با بخش حقیقی مثبت را انتخاب کرد (یا بخش موهومی مثبت، اگر خود آن، عدد حقیقی منفی بود).
با استفاده از رابطهٔ نیم-زاویه
tan
θ
2
=
sin
θ
1
+
cos
θ
{\displaystyle \tan {\frac {\theta }{2}={\frac {\sin \theta }{1+\cos \theta }
arcsin
x
=
2
arctan
x
1
+
1
−
x
2
{\displaystyle \arcsin x=2\arctan {\frac {x}{1+{\sqrt {1-x^{2}
arccos
x
=
2
arctan
1
−
x
2
1
+
x
,
if
−
1
<
x
≤
+
1
{\displaystyle \arccos x=2\arctan {\frac {\sqrt {1-x^{2}{1+x},{\text{ if }-1<x\leq +1}
arctan
x
=
2
arctan
x
1
+
1
+
x
2
{\displaystyle \arctan x=2\arctan {\frac {x}{1+{\sqrt {1+x^{2}
sin
(
arccos
x
)
=
cos
(
arcsin
x
)
=
1
−
x
2
{\displaystyle \sin(\arccos x)=\cos(\arcsin x)={\sqrt {1-x^{2}
sin
(
arctan
x
)
=
x
1
+
x
2
{\displaystyle \sin(\arctan x)={\frac {x}{\sqrt {1+x^{2}
cos
(
arctan
x
)
=
1
1
+
x
2
{\displaystyle \cos(\arctan x)={\frac {1}{\sqrt {1+x^{2}
tan
(
arcsin
x
)
=
x
1
−
x
2
{\displaystyle \tan(\arcsin x)={\frac {x}{\sqrt {1-x^{2}
tan
(
arccos
x
)
=
1
−
x
2
x
{\displaystyle \tan(\arccos x)={\frac {\sqrt {1-x^{2}{x}
تابعهای مثلثاتی در مجموعهٔ اعداد حقیقی، همگی تابعهای متناوب اند و در بازههایی به اندازهٔ ۲π مقدار همهٔ آنها مرتب تکرار میشود. دورهٔ تناوب تابعهای سینوس و کسکانت از ۲πk − π/۲ (به ازای kهای عضو مجموعهٔ اعداد صحیح) شروع میشود و در ۲πk + π/۲ تمام میشود، در نتیجه مقدار تابع میان بازهٔ ۲πk + π/۲ تا ۲πk + ۳π/۲ دوباره بر روی خودش باز میگردد. دورهٔ تناوب کسینوس و سکانت از ۲πk شروع میشود و در ۲πk + π تمام میشود و مقدار تابع در فاصلهٔ میان ۲πk + π تا ۲πk + ۲π دوباره بر روی خودش باز میگردد. دورهٔ تناوب تانژانت از ۲πk − π/۲ شروع میشود و در ۲πk + π/۲ تمام میشود و مقدار تابع به ازای بازههای ۲πk + π/۲ تا ۲πk + ۳π/۲ مرتب تکرار میشود. دورهٔ تناوب کتانژانت از ۲πk شروع میشود و در ۲πk + π تمام میشود، و تابع به ازای بازههای ۲πk + π تا ۲πk + ۲π بر روی خودش باز میگردد.
این تناوب در تابعهای وارون نیز به همین ترتیب ادامه دارد، با فرض اینکه k عدد صحیحی است داریم:
sin
(
y
)
=
x
⇔
y
=
arcsin
(
x
)
+
2
k
π
or
y
=
π
−
arcsin
(
x
)
+
2
k
π
{\displaystyle \sin(y)=x\ \Leftrightarrow \ y=\arcsin(x)+2k\pi {\text{ or }y=\pi -\arcsin(x)+2k\pi }
cos
(
y
)
=
x
⇔
y
=
arccos
(
x
)
+
2
k
π
or
y
=
2
π
−
arccos
(
x
)
+
2
k
π
{\displaystyle \cos(y)=x\ \Leftrightarrow \ y=\arccos(x)+2k\pi {\text{ or }y=2\pi -\arccos(x)+2k\pi }
tan
(
y
)
=
x
⇔
y
=
arctan
(
x
)
+
k
π
{\displaystyle \tan(y)=x\ \Leftrightarrow \ y=\arctan(x)+k\pi }
cot
(
y
)
=
x
⇔
y
=
arccot
(
x
)
+
k
π
{\displaystyle \cot(y)=x\ \Leftrightarrow \ y=\operatorname {arccot}(x)+k\pi }
sec
(
y
)
=
x
⇔
y
=
arcsec
(
x
)
+
2
k
π
or
y
=
2
π
−
arcsec
(
x
)
+
2
k
π
{\displaystyle \sec(y)=x\ \Leftrightarrow \ y=\operatorname {arcsec}(x)+2k\pi {\text{ or }y=2\pi -\operatorname {arcsec}(x)+2k\pi }
csc
(
y
)
=
x
⇔
y
=
arccsc
(
x
)
+
2
k
π
or
y
=
π
−
arccsc
(
x
)
+
2
k
π
{\displaystyle \csc(y)=x\ \Leftrightarrow \ y=\operatorname {arccsc}(x)+2k\pi {\text{ or }y=\pi -\operatorname {arccsc}(x)+2k\pi }
مقالهٔ اصلی: مشتق تابعهای مثلثاتی
مشتق ساده این نوع تابعها، به ازای x های مختلط و حقیقی به قرار زیر است:
d
d
x
arcsin
x
=
1
1
−
x
2
d
d
x
arccos
x
=
−
1
1
−
x
2
d
d
x
arctan
x
=
1
1
+
x
2
d
d
x
arccot
x
=
−
1
1
+
x
2
d
d
x
arcsec
x
=
1
x
x
2
−
1
d
d
x
arccsc
x
=
−
1
x
x
2
−
1
{\displaystyle {\begin{aligned}{\frac {d}{dx}\arcsin x&{}={\frac {1}{\sqrt {1-x^{2}\\{\frac {d}{dx}\arccos x&{}={\frac {-1}{\sqrt {1-x^{2}\\{\frac {d}{dx}\arctan x&{}={\frac {1}{1+x^{2}\\{\frac {d}{dx}\operatorname {arccot} x&{}={\frac {-1}{1+x^{2}\\{\frac {d}{dx}\operatorname {arcsec} x&{}={\frac {1}{x\,{\sqrt {x^{2}-1}\\{\frac {d}{dx}\operatorname {arccsc} x&{}={\frac {-1}{x\,{\sqrt {x^{2}-1}\end{aligned}
رابطههای زیر ویژهٔ x های حقیقی است:
d
d
x
arcsec
x
=
1
|
x
|
x
2
−
1
;
|
x
|
>
1
d
d
x
arccsc
x
=
−
1
|
x
|
x
2
−
1
;
|
x
|
>
1
{\displaystyle {\begin{aligned}{\frac {d}{dx}\operatorname {arcsec} x&{}={\frac {1}{|x|\,{\sqrt {x^{2}-1};\qquad |x|>1\\{\frac {d}{dx}\operatorname {arccsc} x&{}={\frac {-1}{|x|\,{\sqrt {x^{2}-1};\qquad |x|>1\end{aligned}
برای مشتق ساده اگر
θ
=
arcsin
x
{\displaystyle \theta =\arcsin x\!}
d
arcsin
x
d
x
=
d
θ
d
sin
θ
=
1
cos
θ
=
1
1
−
sin
2
θ
=
1
1
−
x
2
{\displaystyle {\frac {d\arcsin x}{dx}={\frac {d\theta }{d\sin \theta }={\frac {1}{\cos \theta }={\frac {1}{\sqrt {1-\sin ^{2}\theta }={\frac {1}{\sqrt {1-x^{2}
عبارت انتگرالی برابر با تابعهای وارون مثلثاتی به قرار زیر است:
arcsin
x
=
∫
0
x
1
1
−
z
2
d
z
,
|
x
|
≤
1
arccos
x
=
∫
x
1
1
1
−
z
2
d
z
,
|
x
|
≤
1
arctan
x
=
∫
0
x
1
z
2
+
1
d
z
,
arccot
x
=
∫
x
∞
1
z
2
+
1
d
z
,
arcsec
x
=
∫
1
x
1
z
z
2
−
1
d
z
,
x
≥
1
arcsec
x
=
π
+
∫
x
−
1
1
z
z
2
−
1
d
z
,
x
≤
−
1
arccsc
x
=
∫
x
∞
1
z
z
2
−
1
d
z
,
x
≥
1
arccsc
x
=
∫
−
∞
x
1
z
z
2
−
1
d
z
,
x
≤
−
1
{\displaystyle {\begin{aligned}\arcsin x&{}=\int _{0}^{x}{\frac {1}{\sqrt {1-z^{2}\,dz,\qquad |x|\leq 1\\\arccos x&{}=\int _{x}^{1}{\frac {1}{\sqrt {1-z^{2}\,dz,\qquad |x|\leq 1\\\arctan x&{}=\int _{0}^{x}{\frac {1}{z^{2}+1}\,dz,\\\operatorname {arccot} x&{}=\int _{x}^{\infty }{\frac {1}{z^{2}+1}\,dz,\\\operatorname {arcsec} x&{}=\int _{1}^{x}{\frac {1}{z{\sqrt {z^{2}-1}\,dz,\qquad x\geq 1\\\operatorname {arcsec} x&{}=\pi +\int _{x}^{-1}{\frac {1}{z{\sqrt {z^{2}-1}\,dz,\qquad x\leq -1\\\operatorname {arccsc} x&{}=\int _{x}^{\infty }{\frac {1}{z{\sqrt {z^{2}-1}\,dz,\qquad x\geq 1\\\operatorname {arccsc} x&{}=\int _{-\infty }^{x}{\frac {1}{z{\sqrt {z^{2}-1}\,dz,\qquad x\leq -1\end{aligned}
مانند تابع سینوس و کسینوس، وارون این توابع را نیز میتوان به کمک سریهای نامتناهی محاسبه کرد:
arcsin
z
=
z
+
(
1
2
)
z
3
3
+
(
1
⋅
3
2
⋅
4
)
z
5
5
+
(
1
⋅
3
⋅
5
2
⋅
4
⋅
6
)
z
7
7
+
⋯
=
∑
n
=
0
∞
(
(
2
n
)
!
2
2
n
(
n
!
)
2
)
z
2
n
+
1
(
2
n
+
1
)
;
|
z
|
≤
1
{\displaystyle {\begin{aligned}\arcsin z&{}=z+\left({\frac {1}{2}\right){\frac {z^{3}{3}+\left({\frac {1\cdot 3}{2\cdot 4}\right){\frac {z^{5}{5}+\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}\right){\frac {z^{7}{7}+\cdots \\&{}=\sum _{n=0}^{\infty }\left({\frac {(2n)!}{2^{2n}(n!)^{2}\right){\frac {z^{2n+1}{(2n+1)};\qquad |z|\leq 1\end{aligned}
arccos
z
=
π
2
−
arcsin
z
=
π
2
−
(
z
+
(
1
2
)
z
3
3
+
(
1
⋅
3
2
⋅
4
)
z
5
5
+
(
1
⋅
3
⋅
5
2
⋅
4
⋅
6
)
z
7
7
+
⋯
)
=
π
2
−
∑
n
=
0
∞
(
(
2
n
)
!
2
2
n
(
n
!
)
2
)
z
2
n
+
1
(
2
n
+
1
)
;
|
z
|
≤
1
{\displaystyle {\begin{aligned}\arccos z&{}={\frac {\pi }{2}-\arcsin z\\&{}={\frac {\pi }{2}-(z+\left({\frac {1}{2}\right){\frac {z^{3}{3}+\left({\frac {1\cdot 3}{2\cdot 4}\right){\frac {z^{5}{5}+\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}\right){\frac {z^{7}{7}+\cdots )\\&{}={\frac {\pi }{2}-\sum _{n=0}^{\infty }\left({\frac {(2n)!}{2^{2n}(n!)^{2}\right){\frac {z^{2n+1}{(2n+1)};\qquad |z|\leq 1\end{aligned}
arctan
z
=
z
−
z
3
3
+
z
5
5
−
z
7
7
+
⋯
=
∑
n
=
0
∞
(
−
1
)
n
z
2
n
+
1
2
n
+
1
;
|
z
|
≤
1
z
≠
i
,
−
i
{\displaystyle {\begin{aligned}\arctan z&{}=z-{\frac {z^{3}{3}+{\frac {z^{5}{5}-{\frac {z^{7}{7}+\cdots \\&{}=\sum _{n=0}^{\infty }{\frac {(-1)^{n}z^{2n+1}{2n+1};\qquad |z|\leq 1\qquad z\neq i,-i\end{aligned}
arccot
z
=
π
2
−
arctan
z
=
π
2
−
(
z
−
z
3
3
+
z
5
5
−
z
7
7
+
⋯
)
=
π
2
−
∑
n
=
0
∞
(
−
1
)
n
z
2
n
+
1
2
n
+
1
;
|
z
|
≤
1
z
≠
i
,
−
i
{\displaystyle {\begin{aligned}\operatorname {arccot} z&{}={\frac {\pi }{2}-\arctan z\\&{}={\frac {\pi }{2}-(z-{\frac {z^{3}{3}+{\frac {z^{5}{5}-{\frac {z^{7}{7}+\cdots )\\&{}={\frac {\pi }{2}-\sum _{n=0}^{\infty }{\frac {(-1)^{n}z^{2n+1}{2n+1};\qquad |z|\leq 1\qquad z\neq i,-i\end{aligned}
arcsec
z
=
arccos
(
1
/
z
)
=
π
2
−
(
z
−
1
+
(
1
2
)
z
−
3
3
+
(
1
⋅
3
2
⋅
4
)
z
−
5
5
+
(
1
⋅
3
⋅
5
2
⋅
4
⋅
6
)
z
−
7
7
+
⋯
)
=
π
2
−
∑
n
=
0
∞
(
(
2
n
)
!
2
2
n
(
n
!
)
2
)
z
−
(
2
n
+
1
)
(
2
n
+
1
)
;
|
z
|
≥
1
{\displaystyle {\begin{aligned}\operatorname {arcsec} z&{}=\arccos {(1/z)}\\&{}={\frac {\pi }{2}-(z^{-1}+\left({\frac {1}{2}\right){\frac {z^{-3}{3}+\left({\frac {1\cdot 3}{2\cdot 4}\right){\frac {z^{-5}{5}+\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}\right){\frac {z^{-7}{7}+\cdots )\\&{}={\frac {\pi }{2}-\sum _{n=0}^{\infty }\left({\frac {(2n)!}{2^{2n}(n!)^{2}\right){\frac {z^{-(2n+1)}{(2n+1)};\qquad \left|z\right|\geq 1\end{aligned}
arccsc
z
=
arcsin
(
1
/
z
)
=
z
−
1
+
(
1
2
)
z
−
3
3
+
(
1
⋅
3
2
⋅
4
)
z
−
5
5
+
(
1
⋅
3
⋅
5
2
⋅
4
⋅
6
)
z
−
7
7
+
⋯
=
∑
n
=
0
∞
(
(
2
n
)
!
2
2
n
(
n
!
)
2
)
z
−
(
2
n
+
1
)
2
n
+
1
;
|
z
|
≥
1
{\displaystyle {\begin{aligned}\operatorname {arccsc} z&{}=\arcsin {(1/z)}\\&{}=z^{-1}+\left({\frac {1}{2}\right){\frac {z^{-3}{3}+\left({\frac {1\cdot 3}{2\cdot 4}\right){\frac {z^{-5}{5}+\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}\right){\frac {z^{-7}{7}+\cdots \\&{}=\sum _{n=0}^{\infty }\left({\frac {(2n)!}{2^{2n}(n!)^{2}\right){\frac {z^{-(2n+1)}{2n+1};\qquad \left|z\right|\geq 1\end{aligned}
همچنین لئونارد اویلر برای وارون تانژانت، سری کارآمدتری را پیدا کرد، که عبارت است از:
arctan
z
=
z
1
+
z
2
∑
n
=
0
∞
∏
k
=
1
n
2
k
z
2
(
2
k
+
1
)
(
1
+
z
2
)
.
{\displaystyle \arctan z={\frac {z}{1+z^{2}\sum _{n=0}^{\infty }\prod _{k=1}^{n}{\frac {2kz^{2}{(2k+1)(1+z^{2})}.}
هشدار : به ازای n = ۰ عبارت به یک ضرب تهی تبدیل میشود که خود برابر با ۱ است.
همچنین در ادامه میتوان نشان داد که:
arctan
z
=
∑
n
=
0
∞
2
2
n
(
n
!
)
2
(
2
n
+
1
)
!
z
2
n
+
1
(
1
+
z
2
)
n
+
1
{\displaystyle \arctan z=\sum _{n=0}^{\infty }{\frac {2^{\,2n}\,(n!)^{2}{\left(2n+1\right)!}\;{\frac {z^{\,2n+1}{\left(1+z^{2}\right)^{n+1}
برای تمامی x های حقیقی و مختلط، رابطههای زیر برقرار است:
∫
arcsin
x
d
x
=
x
arcsin
x
+
1
−
x
2
+
C
∫
arccos
x
d
x
=
x
arccos
x
−
1
−
x
2
+
C
∫
arctan
x
d
x
=
x
arctan
x
−
1
2
ln
(
1
+
x
2
)
+
C
∫
arccot
x
d
x
=
x
arccot
x
+
1
2
ln
(
1
+
x
2
)
+
C
∫
arcsec
x
d
x
=
x
arcsec
x
−
ln
(
x
(
1
+
x
2
−
1
x
2
)
)
+
C
∫
arccsc
x
d
x
=
x
arccsc
x
+
ln
(
x
(
1
+
x
2
−
1
x
2
)
)
+
C
{\displaystyle {\begin{aligned}\int \arcsin x\,dx&{}=x\,\arcsin x+{\sqrt {1-x^{2}+C\\\int \arccos x\,dx&{}=x\,\arccos x-{\sqrt {1-x^{2}+C\\\int \arctan x\,dx&{}=x\,\arctan x-{\frac {1}{2}\ln \left(1+x^{2}\right)+C\\\int \operatorname {arccot} x\,dx&{}=x\,\operatorname {arccot} x+{\frac {1}{2}\ln \left(1+x^{2}\right)+C\\\int \operatorname {arcsec} x\,dx&{}=x\,\operatorname {arcsec} x-\ln \left(x\left(1+{\sqrt {x^{2}-1} \over x^{2}\right)\right)+C\\\int \operatorname {arccsc} x\,dx&{}=x\,\operatorname {arccsc} x+\ln \left(x\left(1+{\sqrt {x^{2}-1} \over x^{2}\right)\right)+C\end{aligned}
تنها برای x ≥ ۱ که عضو مجموعه اعداد حقیقی اند:
∫
arcsec
x
d
x
=
x
arcsec
x
−
ln
(
x
+
x
2
−
1
)
+
C
∫
arccsc
x
d
x
=
x
arccsc
x
+
ln
(
x
+
x
2
−
1
)
+
C
{\displaystyle {\begin{aligned}\int \operatorname {arcsec} x\,dx&{}=x\,\operatorname {arcsec} x-\ln \left(x+{\sqrt {x^{2}-1}\right)+C\\\int \operatorname {arccsc} x\,dx&{}=x\,\operatorname {arccsc} x+\ln \left(x+{\sqrt {x^{2}-1}\right)+C\end{aligned}
تمامی رابطههای بالا به کمک انتگرالگیری جزء به جزء قابل دستیابی است.
با استفاده از
∫
u
d
v
=
u
v
−
∫
v
d
u
{\displaystyle \int u\,\mathrm {d} v=uv-\int v\,\mathrm {d} u}
u
=
arcsin
x
d
v
=
d
x
d
u
=
d
x
1
−
x
2
v
=
x
{\displaystyle {\begin{aligned}u&{}=&\arcsin x&\quad \quad \mathrm {d} v=\mathrm {d} x\\\mathrm {d} u&{}=&{\frac {\mathrm {d} x}{\sqrt {1-x^{2}&\quad \quad {}v=x\end{aligned}
آنگاه:
∫
arcsin
(
x
)
d
x
=
x
arcsin
x
−
∫
x
1
−
x
2
d
x
{\displaystyle \int \arcsin(x)\,\mathrm {d} x=x\arcsin x-\int {\frac {x}{\sqrt {1-x^{2}\,\mathrm {d} x}
با استفاده از تغییر متغیر:
k
=
1
−
x
2
.
{\displaystyle k=1-x^{2}.\,}
پس:
d
k
=
−
2
x
d
x
{\displaystyle \mathrm {d} k=-2x\,\mathrm {d} x}
و
∫
x
1
−
x
2
d
x
=
−
1
2
∫
d
k
k
=
−
k
{\displaystyle \int {\frac {x}{\sqrt {1-x^{2}\,\mathrm {d} x=-{\frac {1}{2}\int {\frac {\mathrm {d} k}{\sqrt {k}=-{\sqrt {k}
دوباره x را جایگزین میکنیم:
∫
arcsin
(
x
)
d
x
=
x
arcsin
x
+
1
−
x
2
+
C
{\displaystyle \int \arcsin(x)\,\mathrm {d} x=x\arcsin x+{\sqrt {1-x^{2}+C}
↑ واژههای مصوّب فرهنگستان تا پایان دفتر دهم فرهنگ واژههای مصوّب