Uniforme verdeling (continu)

Uniforme verdeling (continu)
Kansdichtheid
Verdelingsfunctie
Parameters	${\displaystyle a,b\in (-\infty ,\infty )}$
Drager	${\displaystyle a\leq x\leq b}$
Kansdichtheid	${\displaystyle {\begin{cases}{\frac {1}{b-a}&{\text{voor }a<x<b\\0&{\text{voor}\ x\leq a\ {\text{of }x\geq b\end{cases}$
Verdelingsfunctie	${\displaystyle {\begin{cases}0&{\text{voor }x<a\\{\frac {x-a}{b-a}&{\text{voor }a\leq x<b\\1&{\text{voor }x\geq b\end{cases}$
Verwachtingswaarde	${\displaystyle {\frac {a+b}{2}$
Mediaan	${\displaystyle {\frac {a+b}{2}$
Modus	N/A
Variantie	${\displaystyle {\frac {(b-a)^{2}{12}$
Scheefheid	${\displaystyle 0}$
Kurtosis	${\displaystyle -{\frac {6}{5}$
Entropie	${\displaystyle \ln(b-a)}$
Moment- genererende functie	${\displaystyle {\frac {e^{tb}-e^{ta}{t(b-a)}$
Karakteristieke functie	${\displaystyle {\frac {e^{itb}-e^{ita}{it(b-a)}$
Portaal    Wiskunde

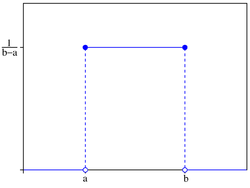
De continue uniforme verdeling is een verdeling op een interval met constante kansdichtheid, wat inhoudt dat er geen voorkeur is voor enige waarde uit dat interval. De kansdichtheid ${\displaystyle f}$ van de uniforme verdeling op het interval ${\displaystyle (a,b)}$ is daarom constant en wordt gegeven door:

${\displaystyle f(x)={\begin{cases}{\frac {1}{b-a}&{\text{voor }a<x<b\\\\0&{\text{elders}\end{cases}$

Voor elk deelinterval ${\displaystyle (x,x+\Delta )}$ met lengte ${\displaystyle \Delta }$ is de kans op een waarde daaruit ${\displaystyle \Delta /(b-a)}$.

De uniforme verdeling kan ook beschouwd worden op half open of gesloten intervallen. De functiewaarden van de dichtheid in de eindpunten van het interval doen niet ter zake. In alle gevallen is de verdelingsfunctie dezelfde:

${\displaystyle F(x)={\begin{cases}0&{\text{voor }x<a\\{\frac {x-a}{b-a}&{\text{voor }a\leq x<b\\1&{\text{voor }x\geq b\end{cases}$

De verwachtingswaarde ${\displaystyle \mathrm {E} X}$ van een uniform op ${\displaystyle (a,b)}$ verdeelde stochastische variabele ${\displaystyle X}$, en de variantie ${\displaystyle \mathrm {var} (X)}$, worden gegeven door:

${\displaystyle \mathrm {E} X={\frac {a+b}{2}$

en

${\displaystyle \mathrm {var} (X)={\frac {(b-a)^{2}{12}$

• Uniforme verdeling (discreet)